Řešení 5-Ox
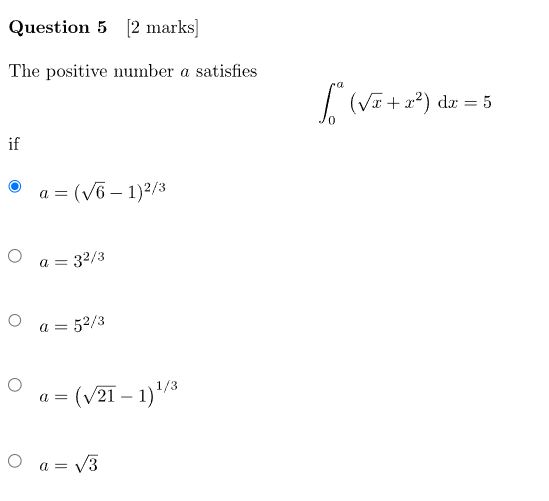
\( \int_0^a{(\sqrt x +x^2)}dx = 5 \)
\( \int_0^a{(\sqrt x +x^2)}dx =\left.\frac{ x^{\frac{3}{2}}} {\frac{3}{2} }+ \frac{x^3}{3} \right|_0^a = \frac{ a^{\frac{3}{2}}} {\frac{3}{2} }+ \frac{a^3}{3} = 5 \)
\( 2a^{\frac{3}{2} }+a^3 =15 \)
\( 4a^3 = (15-a^3)^2 \)
\( 4a^3 =15^2-30a^3+a^6 \)
\(a^6 -34a^3+15^2=0 \)
\( b=a^3\)
\(b^2 -34b+15^2=0 \)
\( b=\frac{34\pm\sqrt{34^2-4\cdot15^2}} {2}\)
\( b=\frac{34\pm\sqrt{256}} {2}=17\pm 8 = 25, 9 \)
\( a=\sqrt[3]{25} =5^{\frac{2}{3}} \)
\( a=\sqrt[3]{9} = 3^{\frac{2}{3}} \)

Řešení 6-Ox
Souřadnice bodu, kde tečna k exponenciele v bode p na protne osu x:
\( y =kx+r \)
\( y =e^px+r \)
\( e^p =e^xp+r \)
\( r=e^p-pe^p=e^p(1-p) \)
\( y =e^px+e^p(1-p) \)
\( 0 =e^px+e^p(1-p) \)
\(0 =x+(1-p) \)
\( a=p-1 \)
\( b=q-1 \)
\( p-a=q-b=1 \)

Řešení 7-Ox
\( e^x=1-e^x \)
\(e^x=\frac{1}{2} \)
\(x=\ln{ \frac{1}{2} } \)
\(x=-\ln{ 2 } \)
\(S =\int_{-\ln{2}}^0 {(e^x-(1-e^x))}dx = \left. {2e^x-x}.\right|_{-\ln{2}}^0 =2-\ln{2}+1=1-\ln{2} \)

Řešení 8-Ox
\( x |x|+1=3 |x| \)
Pro \(x\ge 0 \)
\( x ^2+1=3x \)
\( x ^2-3x+1=0 \)
\( x = \frac{3\pm\sqrt{5}}{2} \) oba dva kořeny jsou větší než 0
Pro \( x < 0 \)
\( -x ^2+1=-3x \)
\( x ^2-3x-1=0 \)
\( x = \frac{3\pm\sqrt{13}}{2} \) pouze jeden kořen je menší než 0
Takže celkem tři kořeny.

